| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | ||
| 6 | 7 | 8 | 9 | 10 | 11 | 12 |
| 13 | 14 | 15 | 16 | 17 | 18 | 19 |
| 20 | 21 | 22 | 23 | 24 | 25 | 26 |
| 27 | 28 | 29 | 30 |
- 제도
- solution
- 치환
- Maths
- GCSE
- differential
- t-치환
- 바이어슈트라스
- Oxford
- division
- equation
- triangle
- mathematics
- 영국
- Order
- a-level
- integral
- factors
- Admissions
- algebraic
- test
- Weierstrass
- DENOMINATOR
- Partial
- 적분
- factor
- fractions
- 교육
- College
- 학년
- Today
- Total
목록수학 모음 (Maths collection) (32)
Cambridge Maths Academy
수학 모음 (Maths collection) 전체보기 This question comes from a test paper for A-level maths for Michaelmas Term at Eton College. Q14. At time $t$ secons after the start of an experiment, the temperature of a cooling liquid is proportional to $\textrm{e}^{-kt}$. (a) Given that the liquid's initial temperature was $80^\circ$C, find a formula for $T$ in terms of $t$ and $k$. [1 mark] (b) Given also that ..
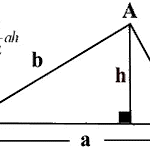 8. Heron's formula - Derivation
8. Heron's formula - Derivation
수학 모음 (Maths collection) 전체보기 Heron's formula calculates the area of a triangle. Here, we will look at two different formulae describing the area of a triangle and then derive the third version, Heron's formula. $A=\frac12ah$ $A=\frac12ab\sin C$ Heron's formula: $A=\sqrt{s(s-a)(s-b)(s-c)}\,$ where $s=\frac{a+b+c}{2}$. s can be thought of as half the perimeter of the triangle. As we will see, the..
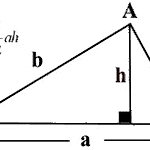 1. 헤론의 공식 유도 (A derivation of Heron's formula)
1. 헤론의 공식 유도 (A derivation of Heron's formula)
헤론의 공식(Heron's formula)은 삼각형의 넓이를 구하는 식이다. 여기서 우리는 삼각형의 넓이를 구하는 두 가지 버전의 공식을 살펴보고, 코사인 법칙(the cosine rule)을 사용하여 헤론의 공식을 유도해보자. $A=\frac12ah$ $A=\frac12ab\sin C$ 헤론의 공식: $A=\sqrt{s(s-a)(s-b)(s-c)}\,$, 그리고 $s=\frac{a+b+c}{2}\,$는 삼각형 둘레의 절반이다. 곧 살펴보겠지만 이 세 가지 공식은 하나의 공식에서 다른 공식을 유도할 수 있는 만큼 모두 동등하다. 따라서 문제에서 주어진 조건에 따라 이 세 가지 공식 중 적절한 것을 선택하여 사용하면 된다. 삼각형의 넓이 1 우리가 가장 먼저 배우는 삼각형의 넓이 공식은 다음과 같다. $$..
수학 모음 (Maths collection) 전체보기 1. $u={\rm csch}\,x+\coth x$ 치환적분 $$ \int{\rm csch}\,x\,\textrm{d}x=-\ln\vert{\rm csch}\,x+\coth x\vert+c $$ 첫 번째 시도로 코시컨트의 적분에서처럼 자연로그를 시도할 수 있다. 쌍곡시컨트(sech)와 달리 쌍곡코시컨트는 삼각함수와 쌍곡선함수의 도함수에 마이너스 차이가 없기 때문에 자연로그 적분이 가능하다는 것을 알게 된다. 우리에게 필요한 도함수들은 다음과 같다. $$ \begin{align} \frac{\textrm{d}}{\textrm{d}x}\csc x&=-\csc x\cot x & \frac{\textrm{d}}{\textrm{d}x}{\rm csch}\,x&..
수학 모음 (Maths collection) 전체보기 0. $\int{\rm sech}\,x\,\textrm{d}x=\ln\vert{\rm sech}\,x+\tanh x\vert+c\,$? 첫 번째 시도로 시컨트의 적분에서처럼 자연로그를 시도할 수 있다. 그러나 삼각함수와 쌍곡선함수의 도함수에서 마이너스 차이로 인해 가능하지 않다는 것을 알게 된다. 이 차이점을 아는 것도 중요하므로 이 부분부터 살펴보자. 우리에게 필요한 도함수들은 다음과 같다. $$ \begin{align} \frac{\textrm{d}}{\textrm{d}x}\tan x&=\sec^2x & \frac{\textrm{d}}{\textrm{d}x}\tanh x&={\rm sech}^2x \\ \frac{\textrm{d}}{\textrm..
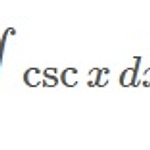 3. 코시컨트/코세칸트(cosec x, csc x)의 적분법 | Integration of cosec x
3. 코시컨트/코세칸트(cosec x, csc x)의 적분법 | Integration of cosec x
코시컨트의 적분은 시컨트와 매우 유사하다. (시컨트 적분의 역사적 배경은 이곳 참조.) 이 글에서는 코시컨트/코세칸트의 적분을 4가지 방법으로 유도해보자. $$ \begin{align} \int \csc x \, \textrm{d}x &= - \ln \vert \csc x + \cot x \vert + c \\ &= \frac12 \ln \left\vert \frac{1-\cos x}{1+\cos x}\right\vert + c \\ &= \ln \left\vert \tan \frac{\pi}{2}\right\vert + c \end{align} $$ 1. $u=\csc x+\cot x$ 치환적분 $$ \int\csc x\,\textrm{d}x=-\ln\vert\csc x+\cot x\vert+c $$ ..
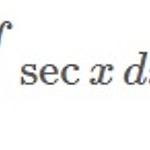 2. 시컨트/세칸트(sec x)의 적분법 | Integration of sec x
2. 시컨트/세칸트(sec x)의 적분법 | Integration of sec x
수학 모음 (Maths collection) 전체보기 0. 역사적 배경 시컨트/세칸트 함수 적분의 역사는 16세기 말~17세기 초, 항해(sea navigation)와 그에 필요한 지도 제작 시기로 거슬러 올라간다. 1569년 벨기에 지도학자/지도작성자(cartographer) 제라두스 메르카토르(Gerardus Mercator, 1512-1594)에 의해 오늘날 '메르카토르 투영법(Mercator projection)'이라고 부르는 지도 작성법이 소개되고, 보다 정확한 계산을 위해 세칸트 함수의 적분법이 필요하게 되었다. (보다 자세한 내용은 논문 참조: History of the Integral of Secant) 1599년 메르카토르 투영법을 염두에 둔 영국 수학자 에드워드 라이트(Edward Wr..
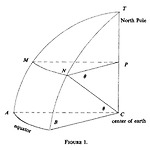 1. History of the Integral of the Secant (sec x)
1. History of the Integral of the Secant (sec x)
수학 모음 (Maths collection) 전체보기 Journal: Mathematics Magazine, volume 53, number 3, May 1980, pages 162–166 Title: An Application of Geography to Mathematics: History of the Integal of the Secant Authors: V. Frederick Rickey (Bowling Green State University, Bowling Green, OH 43403), Philip M. Tuchinsky (Ford Motor Company, Engineering Computing Center, Dearborn, M1 48121) Every student of the inte..
