| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | ||||
| 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| 11 | 12 | 13 | 14 | 15 | 16 | 17 |
| 18 | 19 | 20 | 21 | 22 | 23 | 24 |
| 25 | 26 | 27 | 28 | 29 | 30 | 31 |
- 적분
- factors
- solution
- 바이어슈트라스
- t-치환
- 치환
- differential
- 학년
- Partial
- division
- fractions
- triangle
- Weierstrass
- Admissions
- algebraic
- DENOMINATOR
- 교육
- GCSE
- equation
- Order
- a-level
- College
- Oxford
- test
- integral
- mathematics
- factor
- 영국
- Maths
- 제도
- Today
- Total
Cambridge Maths Academy
P1 §6.4 Use tangent and chord properties 본문
P1 §6.4 Use tangent and chord properties
Cambridge Maths Academy 2020. 6. 14. 23:19Pure mathematics Year 1
Table of contents
- Introduction
- Tangent properties
- Chord properties
- Edexcel P1 Ch6 Exercise 6E
1. Introduction
We can use the properties of tangents and chords within circles to solve geometric problems.
2. Tangent properties
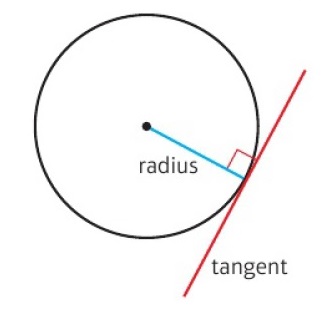
- A tangent to a circle is a straight line that intersects the circle at only one point.
- A tangent to a circle is perpendicular to the radius of the circle at the point of intersection.
Example 1. The circle $C$ has equation $(x-2)^2+(y-6)^2=100$.
(a) Verify that the point $P(10,0)$ lies on $C$.
(b) Find an equation of the tangent to $C$ at the point $(10,0)$, giving your answer in the form $ax+by+c=0$.
Solution.
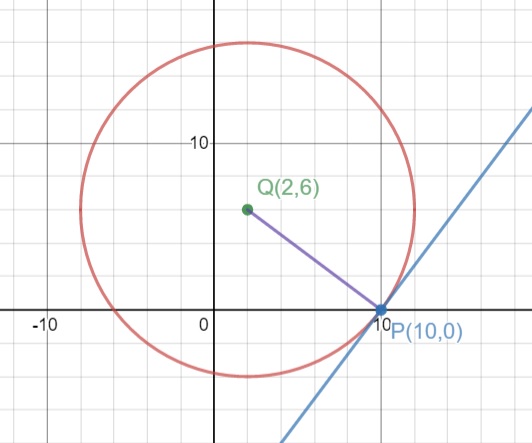
To find the gradient of the tangent, we need to find the gradient of $PQ$:
$$ \begin{align} m=\frac{\Delta y}{\Delta x}=\frac{y_2-y_1}{x_2-x_1}=\frac{0-6}{10-2}=\frac{-6}{8}=-\frac34 \end{align}$$
Since the tangent is perpendicular to $PQ$, its gradient is $\frac43$ and it passes through $P(10,0)$:
$$ \begin{align} y&=\frac43x+c \\ 0&=\frac43\cdot10+c \\ c&=-\frac{40}3 \\ \Rightarrow\qquad y&=\frac43x-\frac{40}3 \\ \Rightarrow\qquad 4x&-3y-40=0 \quad \checkmark \end{align} $$
A similar question may be found in Exercise 6E, Q5.
Example 2. A circle $C$ has equation $(x-5)^2+(y+3)^2=10$.
The line $l$ is a tangent to the circle and has gradient $-3$.
Find two possible equations for $l$, giving your answers in the fom $y=mx+c$.
Solution.
Method 1. We can start with sketching a diagram to understand the situation. We then soon realise that there are two possible tangents with the gradient $-3$ as shown below.
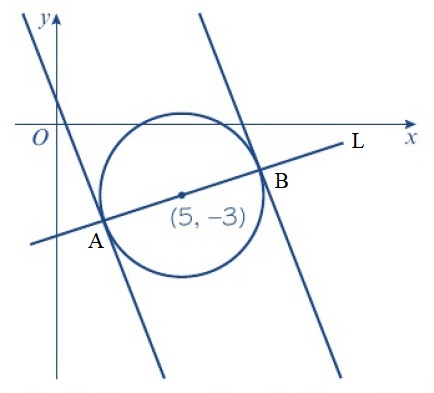
Here's our strategy:
- The line $L$ has gradient $\frac13$ since it is perpendicular to $l$, and it passes through the centre $(5,-3)$. So we can find the equation for $L$: $$ \begin{align} y&=\frac13x+c \\ \textrm{Passing through $(5,-3)$}:\qquad -3&=\frac13\cdot5+c \\ \Rightarrow\qquad c&=3+\frac53=-\frac{14}3 \\ \Rightarrow\qquad y&=\frac13x-\frac{14}3 \end{align} $$
- Now, we can find the points of intersection $A$ and $B$ by solving simultaneous equations: $$ \begin{align} (x-5)^2+(y+3)^2&=10 \\ (x-5)^2+\left(\frac13x-\frac{14}3+3\right)^2&=10 \qquad\left(\textrm{since}\quad L:\quad y=\frac13x-\frac{14}3\right) \\ (x-5)^2+\left(\frac13x-\frac53\right)^2&=10 \\ x^2-10x+25+\left(\frac19x^2-\frac{10}9x+\frac{25}9\right)&=10 \\ \frac{10}9x^2-\frac{100}9x+\frac{160}9&=0 \\ x^2-10x+16&=0 \\ (x-2)(x-8)&=0 \\ \Rightarrow\qquad x=2\quad{\rm or}\quad x&=8 \\ \Rightarrow\qquad y=-4\quad{\rm or}\quad y&=-2 \\ \Rightarrow\qquad A(2,-4)\quad{\rm and}\quad B&(8,-2) \end{align} $$
- and hence the two tangents going through $A$ and $B$ are: $$ \begin{align} \textrm{Tangent through } A(2,-4) \; : && y&=-3x+c_1 \\ && -4&=-3\cdot2+c_1 \\ && c_1&=2 \\ && \Rightarrow \qquad y&=-3x+2 \qquad\checkmark \\ \\ \textrm{Tangent through } B(8,-2) \; : && y&=-3x+c_2 \\ && -2&=-3\cdot8+c_2 \\ && c_2&=22 \\ && \Rightarrow\qquad y&=-3x+22 \qquad\checkmark \end{align} $$
Method 2. Alternatively, we can use the discriminant to find the values of $c$. It is given that $y=-3x+c$ is a tangent to the circle, i.e. the following simultaneous equations has only one solution.
$$ \left\{\begin{array}{l} (x-5)^2+(y+3)^2=10 \\ y=-3x+c \end{array}\right. $$
As we substitute $y=-3x+c$ into the equation of the circle, we find
$$ \begin{align} (x-5)^2+(y+3)^2&=10 \\ (x-5)^2+(-3x+c+3)^2&=10 \\ x^2-10x+25+9x^2-6(c+3)x+(c+3)^2&=10 \\ 10x^2-2[3(c+3)+5]x+(c+3)^2+15&=0 \end{align} $$
Since it has only one solution, its discriminant must be equal to zero, i.e.
$$ \begin{align} \Delta&=b^2-4ac \\ &=4[3(c+3)+5]^2-4\cdot10\cdot\left[(c+3)^2+15\right] \\ &=4\Big[9(c+3)^2+30(c+3)+25-10(c+3)^2-150\Big] \\ &=4\Big[-(c+3)^2+30(c+3)-125\Big] \\ &=-4\Big[(c+3)^2-30(c+3)+125\Big] \\ &=-4(c+3-25)(c+3-5) \\ &=-4(c-22)(c-2) \\ &=0 \end{align} $$ and we find $c=22$ or $c=2$ as before. Thus, the equations of the two tangents are: $$ \begin{align} \left\{ \begin{array}{l} y=-3x+22 \\ y=-3x+2 \end{array} \right. \qquad\checkmark \end{align} $$
A similar question may be found in Exercise 6E, Q7.
Aside. A general case: A circle with equation $$ (x - a)^2 + (x - b)^2 = r^2 $$ The line $l$ is a tangent to the circle and has gradient $m$. Find the two possible equations for $l$ giving in the form $ y = mx + c $.
더보기Method 1.
- Let $L$ be the perpendicular line to $l$ through the centre $(a,b)$. It has gradient $-\frac1m$ since it is perpendicular to $l$. Since it passes through the centre $(a,b)$, we can find its equation: $$ \begin{align} y&=-\frac1mx+c \\ \textrm{Passing through $(a,b)$}:\qquad b&=-\frac1m\cdot a+c \\ \Rightarrow\qquad c&=b+\frac{a}{m}\\ \Rightarrow\qquad y&=-\frac1mx+b+\frac{a}{m} \end{align} $$
- Now, we can find the points of intersection A and B by solving simultaneous equations: $$ \begin{align} (x-a)^2+(y-b)^2&=r^2 \\ (x-a)^2+\left(-\frac1mx+b+\frac{a}{m}-b\right)^2&=r^2 \qquad\left(\textrm{since}\quad L:\quad y=-\frac1mx+b+\frac{a}{m}\right) \\ (x-a)^2+\left( - \frac{ x - a }{m} \right)^2&=r^2 \\ (x-a)^2+ \frac{ (x - a)^2 }{m^2 } &=r^2 \\ \left( \frac{1 + m^2}{m^2} \right) (x - a)^2 &= r^2 \\ (x - a)^2 &= \frac{ r^2 m^2 }{ 1 + m^2 } \\ \Rightarrow \qquad x &= a \pm \frac{rm}{ \sqrt{ 1 + m^2 } } \end{align} $$ and the corresponding $y$-coordinates are: $$ \begin{align} y&= - \frac1m \left( a \pm \frac{rm}{ \sqrt{ 1 + m^2 } } \right) + b + \frac{a}{m} \\ &= b \mp \frac{r}{ \sqrt{ 1 + m^2 } } \end{align} $$ So the points of intersection are: $$ \begin{align} A&=\left( a + \frac{rm}{ \sqrt{ 1 + m^2 } }, b - \frac{r}{ \sqrt{ 1 + m^2 } } \right) \\ B&=\left( a - \frac{rm}{ \sqrt{ 1 + m^2 } }, b + \frac{r}{ \sqrt{ 1 + m^2 } } \right) \end{align} $$
- and hence the two tangents going through $A$ and $B$ are: $$ \begin{align} \textrm{Tangent through } A \; : && y&=mx+c_1 \\ && b - \frac{r}{ \sqrt{ 1 + m^2 } } &= m \left( a + \frac{rm}{ \sqrt{ 1 + m^2 } } \right) + c_1 \\ && c_1 &= b - am - r \sqrt{ 1 + m^2 } \\ && \Rightarrow\qquad y&= mx + b - am - r \sqrt{ 1 + m^2 } \qquad\checkmark \\ \\ \textrm{Tangent through } B\; : && y&=mx+c_2 \\ && b + \frac{r}{ \sqrt{ 1 + m^2 } } &= m \left( a - \frac{rm}{ \sqrt{ 1 + m^2 } } \right) + c_2 \\ && c_1 &= b - am + r \sqrt{ 1 + m^2 } \\ && \Rightarrow\qquad y&= mx + b - am + r \sqrt{ 1 + m^2 } \qquad\checkmark \end{align} $$
Method 2. It is given that $y = mx + c$ is a tangent to the circle, i.e. the following simultaneous equations has only one solution.
$$ \left\{\begin{array}{l} (x-a)^2+(y-b)^2=r^2 \\ y=mx+c \end{array}\right. $$ As we substitute $y=mx+c$ into the equation of the circle, we find $$ \begin{align} (x-a)^2+(mx+c-b)^2&=r^2 \\ (x-a)^2+(mx+c-b)^2&=r^2 \\ x^2 - 2ax + a^2 + m^2 x^2 + 2m (c-b) x + (c-b)^2 &= r^2 \\ \left( 1 + m^2 \right) x^2 + 2 [ m ( c - b ) - a \big] x + (c-b)^2 + a^2 - r^2 &= 0 \end{align} $$ Since it has only one solution, its discriminant must be equal to zero, i.e. $$ \begin{align} \Delta &= 4 \big[ m (c-b) - a \big]^2 - 4 \left( 1 + m^2 \right) \left[ (c-b)^2 + a^2 - r^2 \right] \\ &= 4 \Big( \big[ m (c-b) - a \big]^2 - \left( 1 + m^2 \right) \left[ (c-b)^2 + a^2 - r^2 \right] \Big) \\ &= 4 \Big[ m^2 (c-b)^2 - 2am (c-b) + a^2 - \left( 1 + m^2 \right) (c-b)^2 - \left( 1 + m^2 \right) a^2 + \left( 1 + m^2 \right) r^2 \Big] \\ &= 4 \Big[ - (c-b)^2 - 2am (c-b) - m^2 a^2 + \left( 1 + m^2 \right) r^2 \Big] \\ &= - 4 \Big[ (c-b)^2 + 2am (c-b) + m^2 a^2 - \left( 1 + m^2 \right) r^2 \Big] \\ &= - 4 \Big[ \big[ (c-b) + am \big]^2 - \left( 1 + m^2 \right) r^2 \Big] \\ &=0 \end{align} $$ and thus we find $$ \begin{align} \Rightarrow \qquad c &= b - am \pm r \sqrt{ 1 + m^2 } \end{align} $$ The equations of the two tangents are: $$ \begin{align} \left\{ \begin{array}{l} y = mx + b - am + r \sqrt{ 1 + m^2 } \\ y = mx + b - am - r \sqrt{ 1 + m^2 } \end{array} \right. \qquad\checkmark \end{align} $$
For example,
- $m=0$: the tangents are horizontal lines $$ \begin{align} \left\{ \begin{array}{l} y = b + r \\ y = b - r \end{array} \right. \qquad\checkmark \end{align} $$
- $m=\infty$: the tangents are vertical lines. Rearranging the equation by dividing it by $m$ gives $$ \begin{align} \frac1m y = x + \frac1m b - a \pm \frac{r}{m} \sqrt{ 1 + m^2 } \end{align} $$ As $m \rightarrow \infty$, we find $$ \begin{align} \left\{ \begin{array}{l} x = a + r \\ x = b - r \end{array} \right. \qquad\checkmark \end{align} $$
3. Chord properties
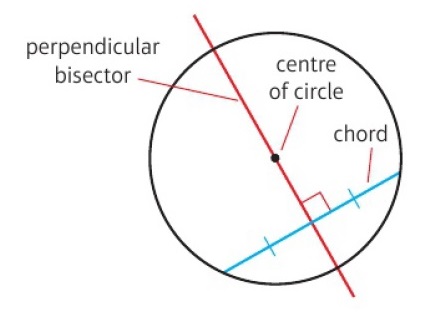
- A chord is a line segment that joins two points on the circumference of a circle.
- The perpendicular bisector of a chord will go through the centre of a circle.
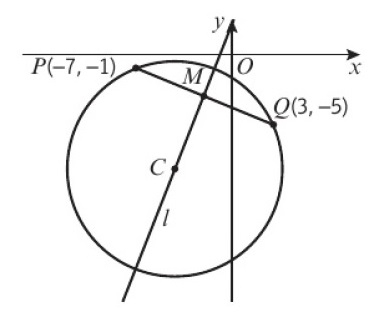
Example 3. The points $P$ and $Q$ lie on a circle with centre $C$, as shown in the diagram.
The point $P$ has coordinates $(-7,-1)$ and the point $Q$ has coordinates $(3,-5)$.
$M$ is the midpoint of the line segment $PQ$.
The line $l$ passes through points $M$ and $C$.
(a) Find an equation for $l$.
Given that the $y$-coordinate of $C$ is $-8$,
(b) show that the $x$-coordinate of $C$ is $-4$
(c) find an equation of the circle.
Solution.
$$ \begin{align} {\rm Gradient\,of\,PQ}=\frac{\Delta y}{\Delta x}=\frac{-5-(-1)}{3-(-7)}=\frac{-4}{10}=-\frac25 \end{align} $$
and hence the gradient of $l$ is $\frac52$. The coordinate of $M$ is given by
$$ \begin{align} M=\left(\frac{x_1+x_2}2,\frac{y_1+y_2}2\right)=\left(\frac{-7+3}2,\frac{-1-5}2\right)=(-2,-3) \end{align} $$
The equation for $l$ is:
$$ \begin{align} y&=\frac52x+c \\ \textrm{Passing through $M(-2,-3)$:}\qquad -3&=\frac52\cdot(-2)+c \\ c&=2 \\ \Rightarrow\qquad y&=\frac52x+2 \end{align} $$
(b) The centre $C$ is on the line $l$ with the $y$-coordinate $-8$, so its $x$-coordinate can be found by the equation for $l$:
$$ -8=\frac52x+2\qquad\Rightarrow\qquad x=\frac25\cdot(-10)=-4 \quad\checkmark $$
(c) Now, to write down the equation for the circle, we need to find the radius and it can be found by either CP or CQ:
$$ \begin{align} CP&=\sqrt{(-7-(-4))^2+(-1-(-8))^2}=\sqrt{(-3)^2+7^2}=\sqrt{9+49}=\sqrt{58} \\ CQ&=\sqrt{(3-(-4))^2+(-5-(-8))^2}=\sqrt{7^2+3^2}=\sqrt{49+9}=\sqrt{58} \end{align} $$
So the equation of the circle is:
$$ (x+4)^2+(y+8)^2=58 \quad\checkmark $$
A similar question may be found in Exercise 6E, Q10.
4. Edexcel P1 Ch6 Exercise 6E
Question 1. The line $ x + 3y - 11 = 0 $ touches the circle $ (x+1)^2 + (y+6)^2 = r^2$ at $(2,3)$.
(a) Find the radius of the circle.
(b) Show that the radius at $(2,3)$ is perpendicular to the line.
Solution.
(a) The point $(2,3)$ is on the circle. $$ \begin{align} r^2 &= (2+1)^2 + (3+6)^2 \\ &= 3^2 + 9^2 \\ &= 90 \\ \Rightarrow \qquad r&= \sqrt{90} = 3\sqrt{10} \end{align} $$
Aside. The fact that the line $ x + 3y - 11 = 0 $ touches the circle: $$ \begin{align} (x+1)^2 + (y+6)^2 &= r^2 \\ (-3y+11+1)^2 + (y+6)^2 &= r^2 \\ 9(y-4)^2 + (y+6)^2 &= r^2 \\ 9 \left( y^2 - 8x + 16 \right) + \left( y^2 + 12y + 36 \right) &= r^2 \\ 10 y^2 - 60 y + 180 - r^2 &= 0 \end{align} $$ requires $\Delta = 0$, i.e. $$ \begin{align} \Delta &= b^2 - 4ac \\ &= 60^2 - 4 \cdot 10 \cdot \left( 180 - r^2 \right) \\ &= 40 \left[ 90 - \left( 180 - r^2 \right) \right] \\ &= 40 \left( r^2 - 90 \right) \\ &=0 \\ \Rightarrow \qquad r&= \sqrt{90} = 3\sqrt{10} \qquad \checkmark \end{align} $$ Now, taking $r^2=90$, we can even find the coordinates of the point of intersection: $$ \begin{align} & \Rightarrow & 10 y^2 - 60 y + 90 &= 0 \\ & \Rightarrow & 10 \left( y^2 - 6y + 9 \right) &= 0 \\ & \Rightarrow & 10 \left( y - 3 \right)^2 &= 0 \\ & \Rightarrow & y&= 3 \end{align} $$ and $$ x = -3y + 11 = -3(3)+11 = 2 $$ and thus the point of intersection is at $(2,3)$.
(b) The line can be written as $$ y = - \frac13 x + \frac{11}{3} $$ The radius at $(2,3)$ is the line segment joining the point with the centre $(-1,-6)$, i.e. its gradient reads $$ \begin{align} \frac{ \Delta y }{ \Delta x } &= \frac{-6-3}{-1-2} = \frac{-9}{-3}=3 \end{align} $$ Since $m_1m_2=-1$, the radius is perpendicular to the line.
Question 2. The point $(1,-2)$ lies on the circle centre $(4,6)$.(a) Find the equation of the circle.
(b) Find the equation of the tangent to the circle at $P$.
Solution.
(a) The centre of the circle is $(4,6)$: $$ (x-4)^2 + (y-6)^2 = r^2 $$ and the circle passes through the point $(1,-2)$: $$ \begin{align} r^2 &= (1-4)^2 + (-2-6)^2 \\ &= (-3)^2 + (-8)^2 \\ &= 9 + 64 \\ &= 73 \end{align} $$ thus the equation of the circle reads $$ (x-4)^2 + (y-6)^2 = 73 $$
(b) The grdient of the radius of the circle at $P(1,-2)$ is: recall the centre $(4,6)$, $$ \begin{align} \frac{ \Delta y }{ \Delta x } = \frac{6-(-2)}{4-1} = \frac{8}{3}. \end{align} $$ Since the tangent at $(1,-2)$ is perpendicular to the radius, the gradient of the tangent is $ - \frac{3}{8}$ and its equation reads $$ y = - \frac{3}{8} x + c $$ Since it passes through $P(1,-2)$, $$ \begin{align} -2 &= - \frac{3}{8} + c \\ \Rightarrow \qquad c &= -2 + \frac{3}{8} = - \frac{13}{8} \end{align} $$ and we find: $$ y = - \frac{3}{8} x - \frac{13}{8} $$
Question 3. The points $A$ and $B$ with coordinates $(-1,-9)$ and $(7,-5)$ lie on the circle $C$ with equation $$ (x - 1)^2 + (y + 3)^2 = 40. $$
(a) Find the equation of the perpendicular bisector of the line segment $AB$.
(b) Show that the perpendicular of bisector $AB$ passes through the centre of the circle $C$.
Solution.
Question 4. (P) The points $P$ and $Q$ with coordinates $(3,1)$ and $(5,-3)$ lie on the circle $C$ with equation $$ x^2 - 4x + y^2 + 4y = 2 $$.
(a) Find the equation of the perpendicular bisector of the line segment $PQ$.
(b) Show that the perpendicular of bisector $PQ$ passes through the centre of the circle $C$.
Solution.
Question 5. (E) The circle $C$ has equation $$ x^2 + 18x + y^2 - 2y + 29 = 0. $$
(a) Verify the point $P(-7,-6)$ lies on $C$. [2 marks]
(b) Find an equation for the tangent to $C$ at the point $P$, giving your answer in the form $ y = mx + c $. [4 marks]
(c) Find the coordinates of $R$, the point of intersection of the tangent and the $y$-axis. [2 marks]
(d) Find the area of the triangle $APR$. [2 marks]
Solution.
Question 6. The tangent to the circle $ (x + 4)^2 + (y - 1)^2 = 242 $ at $(7,-10)$ meets the $y$-axis at $S$ and the $x$-axis at $T$.
(a) Find the coordinates of $S$ and $T$. [5 marks]
(b) Hence, find the area of $ \triangle OST $, where $O$ is the origin. [3 marks]
Solution.
Question 7. The circle $C$ has equation $$ (x + 5)^2 + (y + 3)^2 = 80. $$ The line $l$ is a tangent to the circle and has gradient 2. Find two possible equations for $l$ giving your answers in the form $ y = mx + c $. [8 marks]
Solution.
Method 1. Our logic is:
- Since the gradient of the tangent(s) is given, all we need is a point on the tangent(s).
- $P$ and $Q$ are the points on the two tangents.
- They are also the points of intersection between the line $PQ$ and the circle.
- In order to find their coordinates, we need the equation of the line $PQ$.
So, our strategy is the reverse of these, i.e.
- Find the line $PQ$.
- Find the coordinates of $P$ and $Q$, the points of intersection between the line $PQ$ and the circle.
- Using $P$ and $Q$, we can find the equations of the tangents.
(i) The centre of the circle is at $$ A(-5,-3). $$ Since the line $PQ$ and the tangents are perpendicular, the gradient of $PQ$ is $ - \frac12 $. $$ PQ \; : \qquad y = -\frac12 x + e $$ Since it passes through $ A(-5,-3) $, $$ \begin{align} -3 &= \frac{5}{2} + e \\ \Rightarrow \qquad e &= -3 - \frac{5}{2} = - \frac{11}{2} \end{align} $$ and the equation of the line $PQ$ reads $$ y = -\frac12 x - \frac{11}{2} $$
(ii) Since $P$ and $Q$ are the points of intersection between the circle and the line $PQ$, the coordinates of $P$ and $Q$ are the solutions of the following simultaneous equations, $$ \begin{align} \left\{ \begin{array}{l} (x + 5)^2 + (y + 3)^2 = 80 \\ y = -\frac12 x - \frac{11}{2} \end{array} \right. \end{align} $$ As we substitute the line equation into the circle equation, we find: $$ \begin{align} && (x + 5)^2 + \left( -\frac12 x - \frac{11}{2} + 3 \right)^2 &= 80 \\ &\Rightarrow& (x + 5)^2 + \left( -\frac12 x - \frac{5}{2} \right)^2 &= 80 \\ &\Rightarrow& (x + 5)^2 + \left( - \frac{ x + 5 }{ 2 } \right)^2 &= 80 \\ &\Rightarrow& (x + 5)^2 + \frac{ ( x + 5 )^2 }{ 4 } &= 80 \\ &\Rightarrow& \frac54 (x + 5)^2 &= 80 \\ &\Rightarrow& (x+5)^2 &= 64 \\ &\Rightarrow& x &= -5 \pm 8 = -13 \quad \textrm{or} \quad 3 \end{align} $$ which are the $x$-coordinate of $P$ and $Q$, respectively. To find $y$-coordinates, use the equation of $PQ$: $ y = -\frac12x - \frac{11}{2}$,i.e.
- For $P$: $x = -13$, $$ \begin{align} y = \frac{13}{2} - \frac{11}{2} = 1 \qquad \Rightarrow \qquad P = (-13,1) \end{align} $$
- For $Q$: $x = 3$, $$ \begin{align} y = -\frac{3}{2} - \frac{11}{2} = - \frac{14}{2} = -7 \qquad \Rightarrow \qquad Q = (3,-7) \end{align} $$
(iii) Finally, we can find the equations of the tangents:
- For $l_1$: $y = 2x + c$ passing through $P(-13,1)$. $$ \begin{align} && 1 &= -26 + c \\ &\Rightarrow& c &= 27 \\ \\ &\Rightarrow& y &= 2x + 27 \qquad \checkmark \end{align} $$
- For $l_2$: $y = 2x + d$ passing through $Q(3,-7)$. $$ \begin{align} && -7 &= 6 + d \\ &\Rightarrow& d &= -13 \\ \\ &\Rightarrow& y &= 2x - 13 \qquad \checkmark \end{align} $$
Method 2. We can use the discriminant to find the values of $c$, the $y$-intercept of the tangent. It is given that $y=2x+c$ is a tangent to the circle, i.e. the following simultaneous equations has only one solution.
$$ \left\{\begin{array}{l} (x+5)^2+(y+3)^2=80 \\ y=2x+c \end{array}\right. $$
As we substitute $y=2x+c$ into the equation of the circle, we find
$$ \begin{align} && (x+5)^2+(2x+c+3)^2&=80 \\ &\Rightarrow& x^2 + 10x + 25 + 4x^2 + 4(c+3) x + (c+3)^2 &= 80 \\ &\Rightarrow& 5x^2 + 2 \big[ 2(c+3) + 5 \big] x + (c+3)^2 - 55 &= 0 \\ \end{align} $$
Since it has only one solution, its discriminant must be equal to zero, i.e.
$$ \begin{align} \Delta &=4[2(c+3)+5]^2-4 \cdot 5 \cdot \left[(c+3)^2 =- 55 \right] \\ &=4 \Big[ 4 (c+3)^2 + 20(c+3) + 25 - 5 (c+3)^2 + 275 \Big] \\ &=4 \Big[ -(c+3)^2 + 30(c+3) - 300 \Big] \\ &=-4 \Big[(c+3)^2 - 20(c+3) + 300 \Big] \\ &=-4(c+3-30)(c+3+10) \\ &=-4(c-27)(c+13) \\ &=0 \end{align} $$ and we find $c=27$ or $c=-13$. Thus, the equations of the two tangents are: $$ \begin{align} \left\{ \begin{array}{l} y=2x+27 \\ y=2x-13 \end{array} \right. \qquad\checkmark \end{align} $$
Question 8.
Solution.
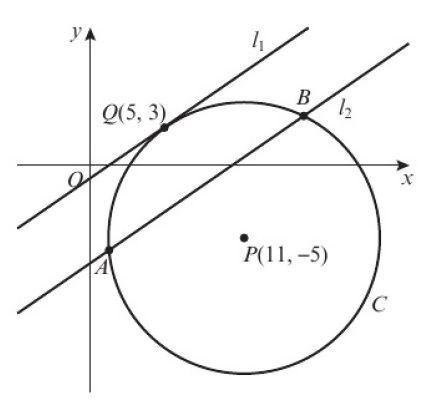
Question 9. (E/P) The circle $C$ has centre $P(11,-5)$ and passes through the point $Q(5,3)$.
(a) Find an equation for $C$. (3 marks)
The line $l_1$ is a tangent to $C$ at the point $Q$.
(b) Find an equation for $l_1$. (4 marks)
The line $l_2$ is parallel to $l_1$ and passes through the midpoint of $PQ$. Given that $l_2$ intersects $C$ at $A$ and $B$
(c) find the coordinates of points $A$ and $B$ (4 marks)
(d) find the length of the line segment $AB$, leaving your answer in its simplest surd form. (3 marks)
Solution.
(a) The centre of the circle is $P(11,-5)$ and the radius is given by $PQ$.
$$ \begin{gather} r=PQ=\sqrt{(5-11)^2+(3-(-5))^2}=\sqrt{(-6)^2+8^2}=10 \\ \therefore\quad (x-11)^2+(y+5)^2=100 \end{gather} $$
(b) The gradient of $PQ$ reads:
$$ \frac{\Delta y}{\Delta x}=\frac{y_2-y_1}{x_2-x_1}=\frac{3-(-5)}{5-11}=\frac{8}{-6}=-\frac43$$
and hence the gradient of $l_1$ is $\frac34$ since it is perpendicular to $PQ$. The equation of the line $l_1$ passing through $Q(5,3)$ is:
$$ \begin{align} y&=\frac34x+c \\ \textrm{Passing through $Q(5,3)$}:\qquad 3&=\frac34\cdot5+c \\ \Rightarrow\qquad c&=-\frac34 \\ \therefore\quad y&=\frac34x-\frac34 \end{align} $$
(c) The midpoint, say $M$, of $PQ$ is given by:
$$ M= \left(\frac{5+11}2,\frac{3-5}2\right)=(8,-1)$$
The equation of the line $l_2$ passing through M is
$$ \begin{align} y&=\frac34x+d \\ \textrm{Passing through $M(8,-1)$}:\qquad -1&=\frac34\cdot8+d \\ \Rightarrow\qquad d&=-7 \\ \Rightarrow\qquad y&=\frac34x-7 \end{align} $$ The points A and B are the points of intersection between the line $l_2$ and the circle $C$, i.e. we solve the simultaneous equations by substituting $l_2$ into $y$ in $C$: $$ \begin{align} (x-11)^2+(y+5)^2&=100 \\ (x-11)^2+\left(\frac34x-7+5\right)^2&=100 \qquad\left(l_2:\quad y=\frac34x-7\right) \\ (x-11)^2+\left(\frac34x-2\right)^2&=100 \\ x^2-22x+121+\left(\frac9{16}x^2-3x+4\right)&=100 \\ \frac{25}{16}x^2-25x+25&=0 \\ x^2-16x+16&=0 \\ (x-8)^2-8^2+16&=0 \\ (x-8)^2&=48 \\ \Rightarrow\qquad x&=8\pm4\sqrt{3} \end{align} $$ The corresponding $y$-values are: $$ \begin{align} y&=\frac34x-7 \\ &=\frac34(8\pm4\sqrt{3})-7 \\ &=6\pm3\sqrt{3}-7 \\ &=-1\pm3\sqrt{3} \end{align} $$ Consider the relative locations of $A$ and $B$, i.e. $x$-coordinate of $B$ is greater than that of $A$, we find $$ \therefore\quad A(8-4\sqrt{3}-1-3\sqrt{3})\qquad{\rm and}\qquad B(8+4\sqrt{3},-1+3\sqrt{3}) $$
(d) The distance $AB$ is given by Pythagoras' theorem:
$$ \begin{align} AB&=\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2} \\ &=\sqrt{\left(8\sqrt{3}\right)^2+\left(6\sqrt{3}\right)^2} \\ &=\sqrt{192+108} \\ &=\sqrt{300} \\ &=10\sqrt{3} \end{align} $$
Question 10.
Solution.
Question 11.
Solution.
Challenge 1.
Solution.
Challenge 2.
Solution.
'A-level Mathematics > Pure Mathematics 1' 카테고리의 다른 글
| P1 §1. Algebraic expressions (0) | 2022.06.30 |
|---|---|
| P1 §8.2 Factorial notation and combination (n choose r, nCr) (0) | 2021.01.12 |
| P1 §8.1 Pascal's triangle (0) | 2021.01.12 |
| P1 §8. The binomial expansion (0) | 2021.01.12 |
| P1 §6. Circles (0) | 2020.06.14 |
